Are men stronger than women?
It sure looks like it. Here are some data from a sample of healthy young
adults. The measure of strength is something called slow, right
extensor, knee peak torque. The dashed lines are drawn through the means
of the men and women at 162 and 99 ft-lbs, respectively (P < 0.001,
Student's t test for independent samples).
One might then ask the question of whether this is still true after adjusting for the fact that men tend to be bigger than women. In other words, ounce for ounce, are women just as strong as men? One way to answer this question is by fitting the analysis of covariance model
where SEX is coded, say, 0 for women and 1 for men. [Since sex can take on only two values, many analyst would prefer the variable name MALE, coded 0 for women and 1 for men, in keeping with the convention that when a variable is named after a "condition", 1 denotes the presence of the condition and 0 denotes its absence. I tend to use variable names like F0M1 where the name contains the codes.] We can use the fitted model to estimate the difference in strength between men and women with the same lean body mass. In this way, the model is said to adjust for lean body mass,
The fitted model is
For a given amount of lean body mass, a male is predicted to be 12.28 ft-lbs stronger than a woman. However, this difference (the coefficient for SEX in the model) is not statistically significant (P = 0.186).
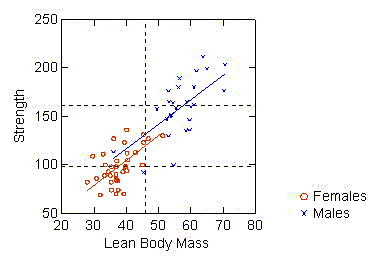
This is illustrated graphically in the scatterplot of strength against lean body mass. Once again, the horizontal dashed lines are drawn through the data at the mean strength values for men and women. We see that men are stronger, but they also have a lot more lean body mass. The red and blue lines are the model fitted to the data. The closeness of the lines suggests that, in this regard at least, men can be thought of as big women or women as small men.
Many investigators like to summarize the data numerically through adjusted means, which take the differences in other variables such as lean body mass into account. Adjusted means are nothing more than the predicted muscle strength of men and women with a given amount of lean body mass. Since the data are consistent with parallel lines, the difference between men and women will be the same whatever the amount of lean body mass. We could report predicted stength for any particular amount of lean body mass without distorting the difference between men and women. Standard practice is to predict muscle strength at the mean value of lean body mass in the combined sample. Here, the mean value of lean body mass is 45.8 kg. Thus, the adjusted mean (strength) for men is 131.3 ft-lb and 119.0 ft-lb for women. These values can be read off the vertical axis by following the vertical line at 45.8 kg of LBM to where it intersects the fitted regression lines.
This example illustrates everything I don't like about adjusted means. In essence, individual adjusted means by themselves don't mean anything! Well, they do, but they may be uninteresting or misleading. These adjusted means arethe estimated strength of a man and woman with 45.8 kg of lean body mass. This is a lot of lean body mass for a women. It's not a lot of lean body mass for a man. It's a value that isn't typical for either group. Those familiar with muscle strength measures might be distracted as they wonder why our men are so weak or women so strong. This gets in the way of the data's message. I find it better to report the constant difference between the two groups.
To be fair, if there is considerable overlap between the groups--that is, if the groups would be expected to be the same with respect to variables being adjusted for if not for the effects of sampling--adjusted means can help bring things back into alignment. However, adjusted means should never be reported without giving critical thought to how they represent the data..